Linear Equations/Slope
Key Skills

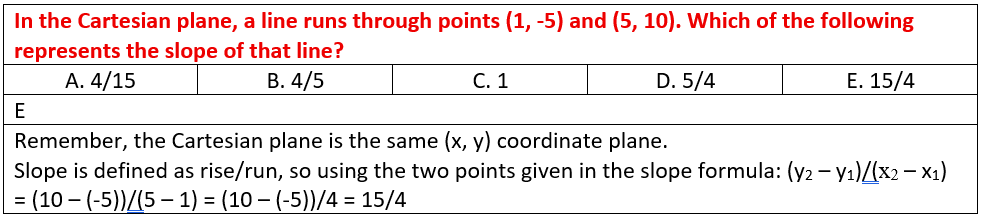
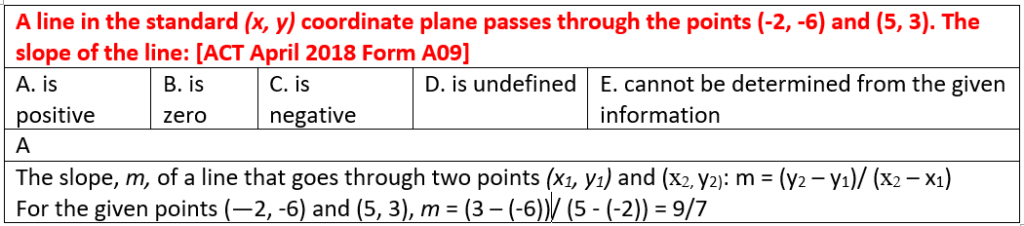
Calculate Slope from Two Points
If given two points on a line, a student should be able to calculate the slope of that line.
The phrase often used to teach this is “rise over run”. Calculate the change in y values and divide it by the change in x values.
(y2 – y1)/(x2 – x1) = m It’s assumed that the a line moves from left to right when calculating slope, so the y2 value should be the y-value of the right-most point, and the x2 value should be the x-value of the right-most point. The other two values then come from the other point.
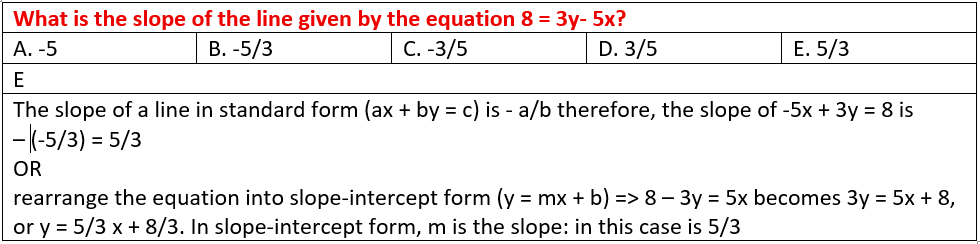
Isolate y (Create y = mx + b)
Sometimes a linear equation will be given in a form other than the classic y = mx + b setup. For these questions, y is treated like any other variable: simply isolate it on the left hand side of the equation.
5x + 5y = 35 becomes x + y = 5 (divide whole equation by 5) and then
y = − x + 5 (−x from both sides)
This is a good reminder that whenever anything shows up on the math section that looks unfamiliar, try out some algebra and see if it can be made to look more familiar. Take the unfamiliar and make it look familiar.
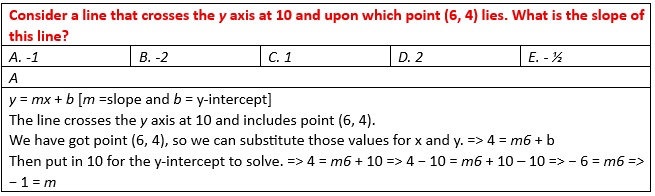
Find Intercepts
The intercepts of a linear equation are where the line hits the x-axis and the y-axis. These are found by setting the opposing variable to zero: equations hit the y-axis/y-intercept when x = 0, equations hit the x-axis/x-intercept when y=0.
For the y-intercept, it’ll be as simple as plugging in 0 for x and then seeing what the output of the equation is. Finding the x-intercept generally requires a little more algebra, as a student works to isolate x.
Key Information for Linear Equations
Perpendicular Slope
When two lines are perpendicular, their slopes are negative reciprocals of each other. That means we can take either slope and find the opposing slope by switching its sign and inverting it between the numerator/denominator.
If there are two lines, m and n, and line m has a linear equation y = 4x + 6, and line n is perpendicular to line m, then it must have a slope of: −1/4.
“Real Solutions”
“Real solution” questions about linear equations will generally start with two linear equations. What matters for these questions are the slopes and intercepts of the given pair of equations. Based on the number of solutions, the following things will be true about the slopes and intercepts of the two lines:
| # of Solutions | Slopes (m) | Intercepts (b) |
|---|---|---|
| 0 | Same | Different |
| 1 | Different | Unknown |
| Infinite | Same | Same |
Real solution questions will either have students calculate the number of solutions for a given pair of equations, or it will state a number of solutions and ask students to create an equation that either satisfies this number of solutions (which one CAN be true) or which cannot satisfy that number of solutions (which one CANNOT be true).
Other keywords to look at for are:
Parallel: Parallel lines have no solutions
Intersecting: Intersecting lines have one solution.
Overlapping: Overlapping lines have infinite solutions.
This is a good reminder that another way to think about solutions with linear equations is as “points of intersection”. The solutions to pairs of equations are where those lines intersect on the xy-plane.

Leave a Reply